Generalized Benford’s Law as a Lie Detector

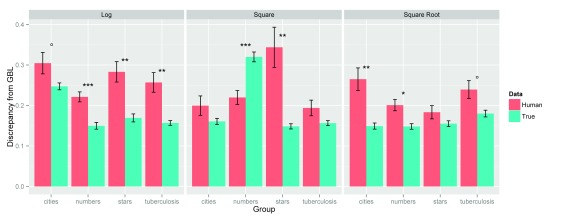
The first significant (leftmost nonzero) digit of seemingly random numbers often appears to conform to a logarithmic distribution, with more 1s than 2s, more 2s than 3s, and so forth, a phenomenon known as Benford’s law. When humans try to produce random numbers, they often fail to conform to this distribution. This feature grounds the so-called Benford analysis, aiming at detecting fabricated data. A generalized Benford’s law (GBL), extending the classical Benford’s law, has been defined recently. In two studies, we provide some empirical support for the generalized Benford analysis, broadening the classical Benford analysis. 

With Benford analysis having become more common in fraud detection, new complementary analyses are needed (Nigrini & Miller, 2009). The GBL analysis potentially provides a whole set of such fraud detection methods, which means making it more difficult, even for informed swindlers intentionally conforming to NBL, to remain undetected.